What is Phi?
Phi ( Φ = 1.618033988749895… ), most often pronounced “fi”, is simply an irrational number like pi ( p = 3.14159265358979… ), but one with many unusual mathematical properties.
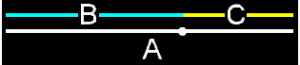
The ratio, or proportion, determined by Phi (1.618 …) was known to the Greeks as the “dividing a line in the extreme and mean ratio” and to Renaissance artists as the “Divine Proportion“ It is also called the Golden Section, Golden Ratio and the Golden Mean. Divide a line so that:
the ratio of the length of the entire line (A)
to the length of larger line segment (B)
is the same as
the ratio of the length of the larger line segment (B)
to the length of the smaller line segment (C).
This happens only at the point where:
A is 1.618 … times B and B is 1.618 … times C.
Alternatively, C is 0.618… of B and B is 0.618… of A.
Phi with an upper case “P” is 1.618 0339 887 …, while phi with a lower case “p” is 0.6180339887, the reciprocal of Phi and also Phi minus 1.
What makes phi even more unusual is that it can be derived in many ways and shows up in relationships throughout the universe.
The Divine Proportion in the Body
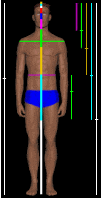
The Divine Proportion in the Body
- The white line is the body’s height.
-
- The blue line, a golden section of the white line, defines the distance from the head to the finger tips.
- The yellow line, a golden section of the blue line, defines the distance from the head to the navel and the elbows.
- The green line, a golden section of the yellow line, defines the distance from the head to the pectorals and inside top of the arms, the width of the shoulders, the length of the forearm and the shin bone.
- The magenta line, a golden section of the green line, defines the distance from the head to the base of the skull and the width of the abdomen. The sectioned portions of the magenta line determine the position of the nose and the hairline.
Although not shown, the golden section of the magenta line (also the short section of the green line) defines the width of the head and half the width of the chest and the hips
The human face is based on Phi and Golden Ratio proportions
- The human face abounds with examples of the Golden Ratio, also known as the Golden Section or Divine Proportion. The head forms a golden rectangle with the eyes at its midpoint. The mouth and nose are each placed at golden sections of the distance between the eyes and the bottom of the chin. The beauty unfolds as you look further.
In animals
The eye, fins and tail all fall at golden sections of the length of a dolphin’s body. The dimensions of the dorsal fin are golden sections (yellow and green). The thickness of the dolphin’s tail section corresponds to same golden section of the line from head to tail.
The eye-like markings of this moth fall at golden sections of the lines that mark its width and length.
The spiral growth of sea shells provide a simple, but beautiful, example.
All the key facial features of the tiger fall at golden sections of the lines defining the length and width of its face.
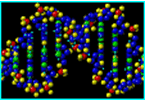
The DNA spiral is a Golden Section
The DNA molecule, the program for all life, is based on the golden section. It measures 34 angstroms long by 21 angstroms wide for each full cycle of its double helix spiral.
34 and 21, of course, are numbers in the Fibonacci series and their ratio, 1.6190476 closely approximates phi, 1.6180339.
Musical scales are based on Fibonacci numbers
The Fibonacci series appears in the foundation of aspects of art, beauty and life. Even music has a foundation in the series, as:
- There are 13 notes in the span of any note through its octave.
- A scale is composed of 8 notes, of which the
- 5th and 3rd notes create the basic foundation of all chords, and
- are based on whole tone which is 2 steps from the root tone, that is the 1st note of the scale.
Note too how the piano keyboard scale of C to C above of 13 keys has 8 white keys and 5 black keys, split into groups of 3 and 2.While some might “note” that there are only 12 “notes” in the scale, if you don’t have a root and octave, a start and an end, you have no means of calculating the gradations in between, so this 13th note as the octave is essential to computing the frequencies of the other notes. The word “octave” comes from the Latin word for 8, referring to the eight whole tones of the complete musical scale, which in the key of C are C-D-E-F-G-A-B-C.
In a scale, the dominant note is the 5th note of the major scale, which is also the 8th note of all 13 notes that comprise the octave. This provides an added instance of Fibonacci numbers in key musical relationships. Interestingly, 8/13 is .61538, which approximates phi. What’s more, the typical three chord song in the key of A is made up
of A, its Fibonacci & phi partner E, and D, to which A bears the same relationship as E does to A. This is analogous to the “A is to B as B is to C” basis for the golden section, or in this case “D is to A as A is to E.”
Musical instrument design is often based on phi, the golden ratio
Automobile logos
The Nissan logo is a simple circle with a cross bar, but the width of that cross bar is defined by perfect golden ratio points of the diameter/height of the circle. The design of the letters is also based on golden ratio proportions, as seen in the horizontal lines in the middle of the “S” and “A.”
The Mazda logo uses the same golden ratio height to width relationship as the Honda logo, with the vertical position of the “M” encircled in the logo also falling roughly at the golden ratio point of the height of the logo.
The Stuttgart emblem in the center of the Porsche logo has a width that is a golden ratio of the midpoint of the width of the shield to the left and right sides. The top of the emblem is at the golden ratio of the midpoint of the height of the shield to the top and bottom.
Phi (Φ), the Golden Section, has been used by mankind for centuries in architecture
Its use started as perhaps early as with the Egyptians in the design of the pyramids. When the basic phi relationships are used to create a right triangle, it forms the dimensions of the great pyramids of Egypt, with the geometry shown below creating an angle of 51.83 degrees, the cosine of which is phi, or 0.618.
The ancient Greek Euclid ((365–300 BC) wrote of it in “Elements” as the ”dividing a line in the extreme and mean ratio.” The Parthenon, built in 447 to 438 BC, appears to use it in some aspects of its design to achieve beauty and balance its design.
Notre Dame in Paris, which was built in between 1163 and 1250 appears to have golden ratio proportions in a number of its key proportions of design. Although it is rather asymmetrical in its design and difficult to measure photographically because of parallax distortions, the golden ratio lines of the green, blue and red rectangles conform closely to the major architectural lines, which represent:
- Red – Vertical height of base at ground level : Top of first level : Top of second floor
- Blue – Vertical height of base of second level : Top of second level : Top of third level
- Green – Horizontal width of outside of left top section : Inside of top right section : Outside of top right section:
Renaissance artists of the 1500′s in the time of Leonardo Da Vinci knew it as the Divine Proportion. In India, it was used in the construction of the Taj Mahal, which was completed in 1648.
SOURCE : GARY MEISNER, WWW.GOLDENNUMBER.NET visit for more information on the golden number and its omnipotency.